Vol. 7 No. 1 (2023)
Research Articles

Equivalence of Electromagnetic Fluctuation and Nuclear (Yukawa) Forces: the π₀ Meson, its Mass and Lifetime

PDF
Editorial History
  • Barry W. Ninham,
  • Iver Brevik,
  • Mathias Boström
Barry W. Ninham
Department of Materials Physics, Research School of Physics, Australian National University, Canberra, Australia
Bio
Iver Brevik
Department of Energy and Process Engineering, Norwegian University of Science and Technology, Trondheim, Norway
Bio
Mathias Boström
University of Oslo
Bio
Adaptation of a Feynman diagram for a To meson. The lines represent the surfaces of two nucleons, protons (p) or neutrons (n), approximated as perfectly reflecting planes. The shade in the intermediate region between the plates represents the high density virtual electron-positron sea from the photons of black body radiation at high temperature (close separation). The meson is a collective excitation (plasmon) in the sea.
DOI: https://doi.org/10.36253/Substantia-1807

Published 2022-12-05

Keywords

  • Casimir-effect,
  • positron-electron-plasma,
  • Meson-theory,
  • lifetime

How to Cite

Ninham, B. W., Brevik, I., & Boström, M. (2022). Equivalence of Electromagnetic Fluctuation and Nuclear (Yukawa) Forces: the π₀ Meson, its Mass and Lifetime. Substantia, 7(1), 7–14. https://doi.org/10.36253/Substantia-1807

Copyright (c) 2022 Barry W. Ninham, Prof. Iver Brevik, Mathias Boström

Creative Commons License

This work is licensed under a Creative Commons Attribution 4.0 International License.

Crossref
Scopus
Google Scholar
Europe PMC

Abstract

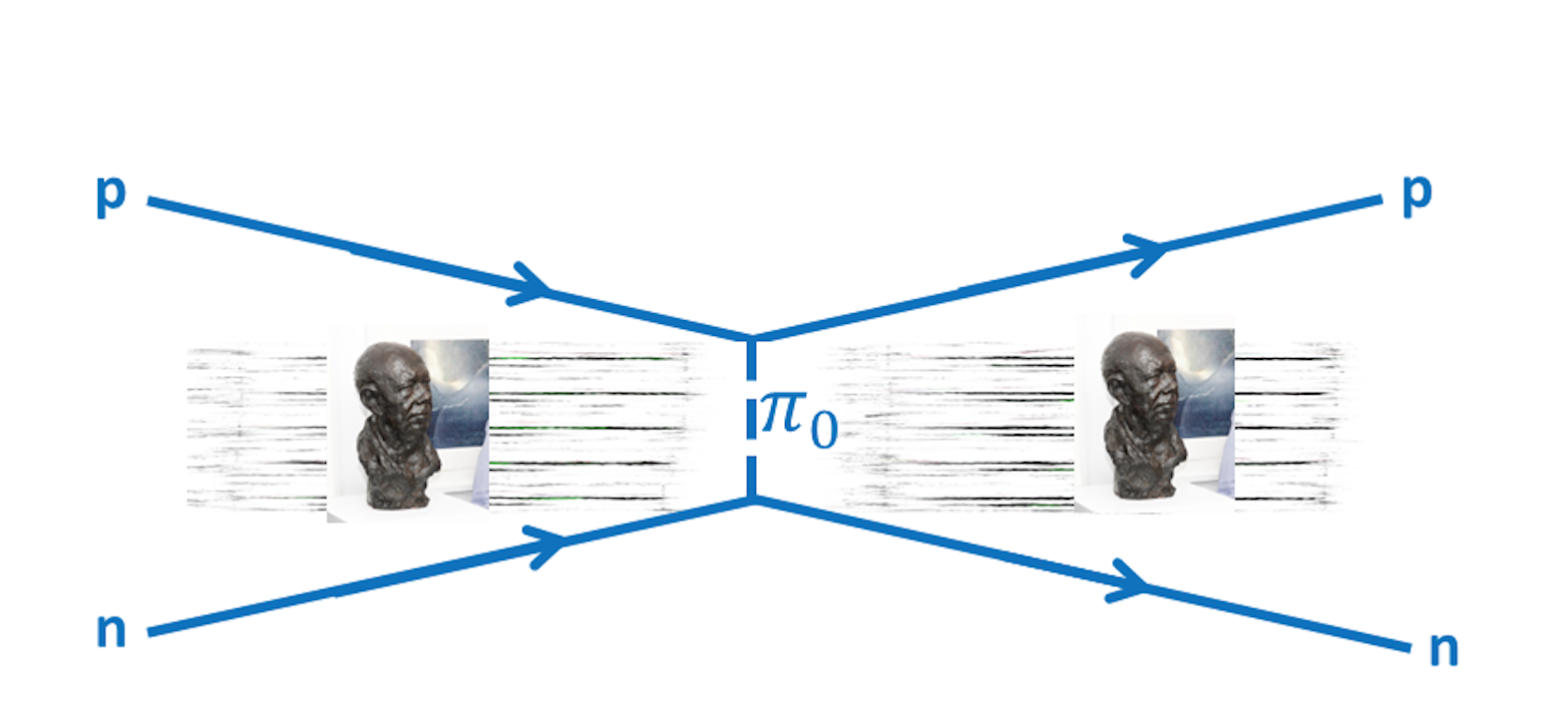
It is shown how Maxwell’s equations for the electromagnetic field with Planck quantisation of allowed modes appears to provide a semiclassical account of nuclear interactions. The mesons emerge as plasmons, collective excitations in an electron positron pair sea. The lifetime and mass of mesons are predicted.

PDF
Editorial History

References

  1. Freeman Dyson, communicated to BWN. See also: F. J. Dyson, ”Feynman’s proof of the Maxwell’s equations”, Am. J. Phys. 58, 209 (1990). A related observation was made by R. P. Feynman, Phys. Rev. 80, 440 (1950): ‘‘high energy potentials could excite states corresponding to other eigenvalues, possibly thereby corresponding to other masses”.
  2. G. C. Wick, “Range of nuclear forces in Yukawa's theory”, Nature 142, 293-294 (1937).
  3. H. B. G. Casimir, “On the attraction between two perfectly conducting plates”, Proc. K. Ned. Akad. Wet. 51, 793 (1948).
  4. B. W. Ninham and V. A. Parsegian, “van der Waals forces: special characteristics in lipid-water systems and a general method of calculation based on the Lifshitz theory”, Biophys. J. 10, 646 (1970).
  5. V. A. Parsegian and B. W. Ninham , “Temperature-dependent van der Waals forces.”, Biophys. J. 10, 664 (1970).
  6. D. J. Mitchell, B. W. Ninham and P. Richmond, “On black body radiation and the attractive force between two metal plates”, Am. J. Phys. 40, 674 (1972).
  7. I. E. Dzyaloshinskii, E. M. Lifshitz, and P. P. Pitaevskii, ”The general theory of van der Waals forces”, Advan. Phys. 10, 165 (1961).
  8. Deryaguin in B.V Deryaguin, I.I . Abrikosova and E. M Lifshitz, ”Direct measurement of molecular attraction between solids separated by a narrow gap”, Quart. Revs. Chem.Soc 10, 295 (1956).
  9. B. W. Ninham, V. A. Parsegian and G. Weiss, “On the macroscopic theory of temperature-dependent van der Waals forces”, J. Stat. Phys. 2, 323 (1970).
  10. P. Richmond and B. W. Ninham, “A note on the extension of the Lifshitz theory of van der Waals forces to magnetic media”, J. Phys. C: Solid State Phys. 4, 1988 (1971).
  11. G. Bimonte, “Beyond-proximity-force-approximation Casimir force between two spheres at finite temperature”, Phys. Rev. D 97, 085011 (2018).
  12. J. Lekner, Theory of Reflection. Reflection and Transmission of Electromagnetic, Particle and Acoustic Waves, 2nd Ed. (Springer, New York, 2016).
  13. E. M. Lifshitz, ”The theory of molecular attractive forces between Solids”, Sov. Phys. 2, 73 (1956).
  14. B. W. Ninham, and J. Daicic, “Lifshitz theory of Casimir forces at finite temperature”, Phys. Rev. A 57, 1870 (1998).
  15. L. D. Landau, E. M. Lifshitz, Statistical Physics, Part 1, 3rd edition (Butterworth-Heinemann, Oxford, 1999).
  16. H. Yukawa, “On the interaction of elementary particles. I”, Proc. Phys. Math. Soc. Japan 17, 48 (1935).
  17. L. D. Landau, E. M. Lifshitz, Quantum Mechanics (Non-relativistic Theory), 3rd edition (Butterworth-Heinemann, Oxford, 1997).
  18. J. Mahanty and B. W. Ninham, Dispersion Forces, (Academic Press, London, 1976).
  19. B. W. Ninham and M. Boström, “Screened Casimir force at finite temperatures: a possible role for nuclear interactions”, Phys. Rev. A 67, 030701 (2003).
  20. B. W. Ninham, M. Boström, C. Persson, I. Brevik, S. Y. Buhmann, and Bo E. Sernelius, ”Casimir forces in a plasma: possible connections to Yukawa potentials”, Eur. Phys. J. D 68, 328 (2014).
  21. P. A. Zyla et al. (Particle Data Group), ”Review of particle physics”, Prog. Theor. Exp. Phys. 2020, 083C01 (2020); page 1212.
  22. B. W. Ninham, C. J. Powell, and N. Swanson, “Plasmon damping in metals”, Phys. Rev. 145, 209 (1966).
  23. S. Weinberg, The Quantum Theory of Fields, Volume II Modern Applications (Cambridge University Press, Cambridge, 2016).
  24. W. E. Burcham and M. Jobes, Nuclear and particle physics, (Longman Scientific & Technical, Essex, 1995).
  25. J. D. Jackson, The Physics of Elementary Particles (Princeton Legacy Library, London, 1958).
  26. I. Larin et al., ”Precision measurement of the neutral pion lifetime”, Science 368, 505 (2020).
  27. H. B. Meyer, ”?_0 decay precision-tests the chiral anomaly”, Science 368, 469 (2020).
  28. K. Kampf and B. Moussallam, ” Chiral expansions of the ?_0 lifetime”, Phys. Rev. D 79, 076005 (2009).
  29. A. M. Bernstein and B. R. Holstein, ”Neutral pion lifetime measurements and the QCD chiral anomaly”, Rev. Mod. Phys. 85, 49 (2013).
  30. J. J. Aubert, et al., ”The ratio of the nucleon structure functions F2N for iron and deuterium”, Phys. Lett. B 123, 275–278 (1983).
  31. G. Feldman, “Origin of neutron and proton changes in nuclei”, Nature 566, 332 (2019).
  32. The CLAS Collaboration, ”Modified structure of protons and neutrons in correlated pairs” Nature 566, 354 (2019).
  33. O. Mauel, C. Bolon, A. Katragada, and M. Insall, ”Attraction and repulsion of nucleons: sources of stellar energy”, J. Fusion Energy 19, 93 (2000).
  34. V. Kowalenko, N. E. Frankel, and K. C. Hines, ”Response theory of particle-anti-particle plasmas”, Phys. Rep. 126, 109 (1985).
  35. J. Daicic, N. E. Frankel, and V. Kowalenko, ”Magnetized pair Bose gas: relativistic superconductor”, Phys. Rev. Lett. 71, 1779 (1993).
  36. J. Daicic, N. E. Frankel, R. M. Gailis, and V. Kowalenko, ”Statistical mechanics of the magnetized pair quantum gases”, Phys. Rep. 237, 128 (1993).
  37. B. W. Ninham and V. V. Yaminsky, “Ion binding and ion specificity: The Hofmeister effect Onsager and Lifschitz theories”, Langmuir 13, 2097 (1997).
  38. B. D. Hughes and B. W. Ninham, ”A correspondence theory”, Physica A 443, 495 (2016). [This publication promotes modern analysis as the fundamental language of quantum physics. A corrected version of this work was published in Substanta 2, 51 (2018)].
  39. D. Parsons, M. Boström, P. Lo Nostro, B. W. Ninham, “Hofmeister effects: interplay of hydration, non-electrostatic potentials, and ion size”, Phys. Chem. Chem. Phys. 13, 12352 (2011).
  40. B. W. Ninham and P. Lo Nostro, Molecular Forces and Self Assembly in Colloid, in Nano Sciences and Biology, (Cambridge University Press, Cambridge, 2010).
  41. M. Boström, D. R. M. Williams, and B. W. Ninham, “Specific ion effects: why DLVO theory fails for biology and colloid systems”, Phys. Rev. Lett. 87, 168103 1-4 (2001).

Most read articles by the same author(s)

1 2 > >>